Calculating the Internal Rate of Return (IRR) for an investment project is an important way to evaluate potential investments. IRR calculates the expected rate of return for a project to determine if the investment is worthwhile. Using Excel to calculate IRR is easy with the built-in IRR function. In this comprehensive guide, we’ll walk through step-by-step how to calculate IRR in Excel.
Understanding IRR and Why It’s Important
The Internal Rate of Return (IRR) is the discount rate that makes the net present value (NPV) of an investment zero. In other words, it is the expected compound annual rate of return for a project.
IRR is commonly used to evaluate the desirability of investments or projects. It accounts for the time value of money – the idea that money available now is worth more than the identical sum in the future due to its potential earning capacity.
Knowing a project’s IRR allows you to quickly determine if it is a good investment. Generally, if the IRR is greater than the cost of capital, the project is considered an attractive investment. Calculating IRR properly is critical for making informed financial decisions.
IRR Calculation Overview
At its core, IRR is the discount rate that makes the net present value equal to zero. NPV calculates the difference between the present value of future cash flows and the initial investment.
Setting NPV equal to zero and solving for the discount rate provides the IRR. This is done through an iterative trial-and-error approach since IRR cannot be calculated directly.
The steps for calculating IRR are:
- Determine the cash flows for the project
- Input the cash flows and initial investment into Excel
- Use Excel’s IRR function to calculate the IRR
Below we’ll go through this process step-by-step.
Step 1: Determine the Investment Cash Flows
The first step is to determine the investment’s cash inflows and outflows over the project’s lifetime. This requires making projections about things like:
- Initial investment amount
- Revenues or cost savings from the project
- Operating and maintenance costs
- Taxes
- Salvage value at the end of the project
Evaluate the investment horizon and make cash flow projections for each period. Be sure to include the initial investment as a negative cash flow. The more detailed and accurate your cash flow projections, the better the IRR calculation.
Step 2: Input the Cash Flows into Excel
Once the cash flow projections are made, input them into an Excel spreadsheet. Place the cash flows into a single column with each row representing a different time period.
For example, the cash flows may look something like this:
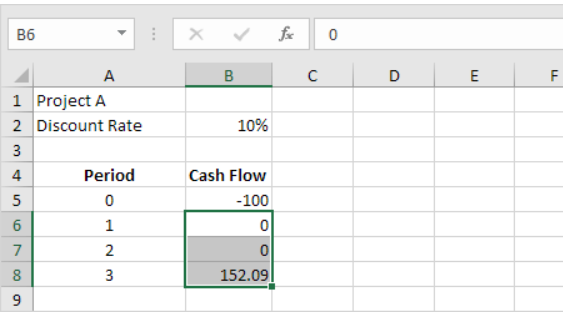
The initial investment is represented by a negative cash flow in period 0. Then the revenues or cost savings for periods 1 through 3 are input as positive values.
Be sure to include a column for the time period and label the rows and columns clearly. Proper labeling will help avoid errors.
Step 3: Use the IRR Function in Excel
Now that the cash flows are entered, it’s time to use Excel’s IRR function. The syntax for the function is:
=IRR(values, [guess])
Where:
Values = the cells containing the cash flows
Guess = (optional) your estimate of what the IRR value is
For example, if the cash flows were in cells B5 through B8, the function would be:
=IRR(B5:B8)
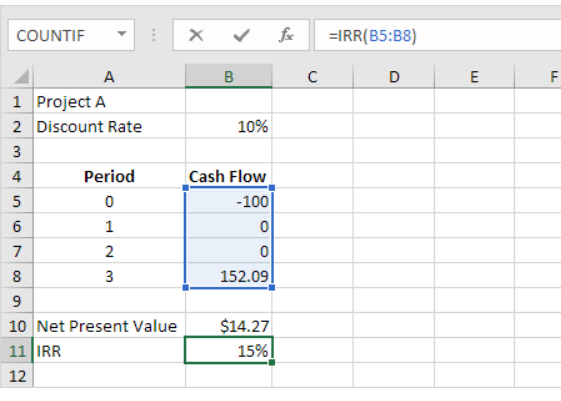
The result is the calculated internal rate of return.
By default, Excel iterates up to 20 times to find the IRR. If it can’t find a result, it will return an error. If this happens, you can provide a guess value as a starting point.
You can also press F2 after entering the function to see the iterations Excel goes through to find the IRR.
The Importance of Cash Flow Order in Excel
One important point about using the IRR function in Excel is that the order of cash flows matters. Excel assumes the first cash flow happens in period 0, then periods 1, 2, 3, and so on, based on the row order. Be sure to enter the cash flows in chronological order in the spreadsheet for an accurate IRR calculation.
Comparing IRR to Cost of Capital
Once the IRR is calculated, it can be compared to the company or project’s cost of capital.
The cost of capital is the minimum return needed on investment – this hurdle rate is often a company’s weighted average cost of capital (WACC).
Comparing IRR to the cost of capital tells you whether the expected return justifies the investment.
- If IRR > Cost of Capital, the project is worthwhile
- If IRR < Cost of Capital, the project should be rejected
For example, if a project has an IRR of 15% and the company’s cost of capital is 12%, the IRR exceeds the minimum return needed so the project is a good investment.
IRR vs NPV for Investment Analysis
While IRR is a helpful metric for evaluating investments, it does have some limitations. It’s also important to look at net present value (NPV).

NPV discounts the cash flows at the cost of capital to give the present value in today’s dollars. It represents the dollar value the investment adds to the firm.
IRR and NPV may sometimes conflict when choosing between mutually exclusive projects. In general, NPV is considered a better indicator since it maximizes the firm’s value. IRR should not be relied on solely.
Using both IRR and NPV together provides a more complete view of an investment’s desirability and potential downsides.
Additional IRR Considerations
Here are some other important notes when using IRR:
- IRR assumes cash flows are reinvested at the IRR rate – this may be unlikely in practice.
- Projects with conventional cash flow patterns (positive then negative) have one IRR. Projects with non-conventional patterns may have multiple IRRs.
- For projects with a short time horizon, modified IRR calculations may be more appropriate.
- IRR does not directly account for project scale or size. Larger projects aren’t necessarily better.
- Using IRR for capital budgeting should take into account factors like project risks and non-monetary benefits.
IRR Calculation Tips
To effectively use IRR in Excel for investment analysis, here are some useful tips:
- Check IRR formula inputs carefully to avoid errors.
- Test different IRR guess values if Excel returns an error.
- Ensure cash flows are in the proper chronological order.
- Use NPV along with IRR to make informed decisions.
- Make detailed, accurate cash flow projections.
- Compare IRR to the appropriate cost of capital benchmark.
Limitations of IRR
Despite its usefulness for investment evaluation, IRR does have some limitations to be aware of:
- Assumes cash flows are reinvested at the IRR – this may not be feasible and overstates the actual return.
- Doesn’t consider project scale – two projects can have the same IRR but differ significantly in size.
- Multiple IRRs are possible – makes comparison difficult for certain project types.
- Focuses on rate of return and doesn’t maximize firm value like NPV.
- Can give unreliable results for long-lived projects.
Understanding these limitations helps ensure IRR is applied properly in investment analysis. Using IRR with other metrics gives a more complete picture.
Conclusion
IRR is an important metric used by businesses to evaluate capital budgeting decisions and potential investments. By calculating a project’s expected internal rate of return, companies can quickly assess if an investment is likely to be profitable.
In Excel, the IRR function makes finding IRR easy and convenient. Just be sure to input the proper cash flows in chronological order to get an accurate result. Comparing the IRR to the cost of capital for a minimum return gives a straightforward assessment of investment desirability.
Used along with other metrics like NPV, IRR can provide significant insights into capital budgeting decisions. It allows companies to quantitatively evaluate investments in a way that accounts for the time value of money. By mastering IRR in Excel, businesses can make smart, informed financial choices to maximize value.
